1)  Self-parallel surface.

A self-parallel surface is a connected surface parallel to itself (at a nonzero distance).

Examples:
    - the sphere is self-parallel with parallelism distance equal to its diameter.
    - the surfaces parallel to a Mobius strip are self-parallel.

See also the self-parallel curves.

2) Surface of constant width.
 

Other name: surface of constant thickness, spheroform (surface). Bibliography: Marc Roux, Ensembles gonfles en dimension n images.math.cnrs.fr/Le-triangle-de-Reuleaux.html T. Bayen, J.-B. Hiriart-Urruty, Objets convexes de largeur constante (en 2D) ou d’epaisseur constante (en 3D) : du neuf avec du vieux. PhD thesis of T. Bayen

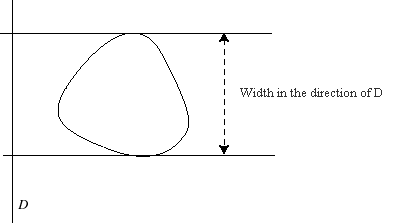
Given a compact convex K of the space, define its width in the direction of the line D as the length of the orthogonal projection of K on D, length which also is the smallest width of a strip orthogonal to D and containing K, or, which amounts to the same thing, the distance between two supporting planes of the convex K that are orthogonal to D.

The convex K is then said to be "of constant width" if this width does not depend on the direction D and a surface is said to be "of constant width" if it is the boundary of a compact convex set of constant width. Another way of defining the fact that K has constant width is that it can take all the different possible positions in the space delimited by two parallel planes so that the two planes always remain in contact with K.

The link with 1) comes from the fact that any convex (i.e. boundary of a convex compact set) self-parallel surface (with parallelism distance d) is a surface of constant width d.

Examples other than the sphere:
 

The surface of revolution generated by the rotation of a Reuleaux triangle around one of its axes of symmetry is a surface of constant width. More generally, any surface of revolution generated by a curve of constant width that has an axis of symmetry is of constant width.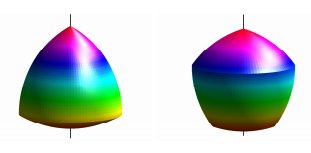
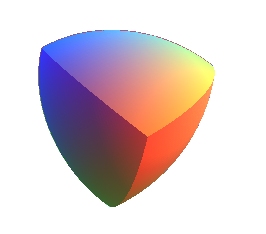
The Reuleaux tetrahedron, composed of four portions of spheres centered on the vertices of a regular tetrahedron is not a surface of constant width. The distance between two points located at the middle of two opposite edges is greater than the distance from a vertex to the opposite face. But the Reuleaux tetrahedron can be modified to become a surface of constant width. See the Meissner solid.

See curves of constant width.
 
 

next surface

previous surface

2D curves

3D curves

surfaces

fractals

polyhedra

© Robert FERRÉOL 2017